The strongest forms of all classes of materials are those that are fibrous in nature. Fibre reinforcement provides dramatic increases in stiffness and strength of polymers, enhanced high temperature properties in metals and improved toughness in ceramics.

Bi-Stable composite shells are straight, thin-walled tubes with a semi/full circular cross-section. They are configurable between two stable states, a coiled state in which the shell is folded along its length and an extended state in which the shell is unfolded. These flexible shells can be folded without permanent deformation and are capable of self deployment through the controlled release of elastic strain energy accumulated during folding. The simplest of this is the steel tape measure, from which variety of concepts have been derived.

These shells can be used for large deployable structures as deployment actuators in a similar manner to tape-springs. But unlike tape-springs, they do not need any drum to roll upon. Among the many other uses to which these bi-stable shells can be put are water pipes, extendible handles and probes, vehicle hoods, roll up ladders, aerial masts, camera mounts, microphone booms, conveyor belts, telecommunications or computer cable ducting and tent poles.

It is found that the shell stretches more along its length than in the transverse length when changes from flattened surface to curved. It is also found that the radius of shell's cross-section in its extended state is almost equal to the inner radius of the shell in its coiled state. A theoretical model is proposed for the total strain energy, including the bending strain energy and membrane strain energy, contained by the shell as a function of the longitudinal curvature, the transverse curvature, the radius of the shell and the angle subtended by the shell's cross-section.

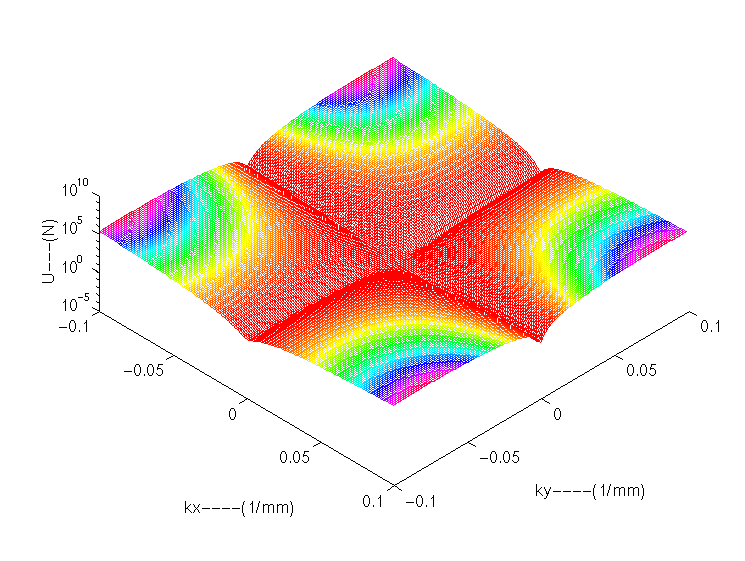
According to this model, the strain energy increases as we move away from the zero energy point 'A'=(0, 1/R), which corresponds to the extended, undtrained configuration. This increase is particularly sensitive to the variations in the longitudinal curvature of the shell and less so to variations in the transverse curvature. In fact, if the imposed curvature changes follow the minimum energy path that is sketched in the figure below, the energy first increases from zero up to the maximum value and then decreases to the local minima, at point 'B'. The configuration corresponding to point 'B' is the second stable, rolled-up configuration of the tube. Experiments, including Tension, bending and torsion tests are performed to characterise the behaviour of PP/Glass laminated composite. The 'laminate theory' is used to calculate the extensional, coupling and bending compliances which are compared to the compliances measured from the experiments.

Last updated on the 13th of April, 1999